|
|
生如夏花(2365*****) 11:29:38
看个数学题
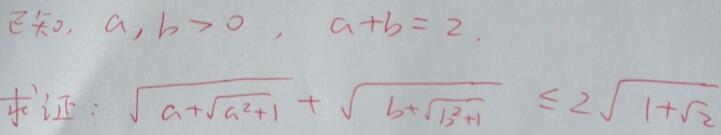
\[f(x)=\sqrt{x+\sqrt{x^2+1}},\quad x\in[0,+\infty),\]
求二阶导数并化简得
\[f''(x)=\frac{(1-3x^2)\sqrt{x+\sqrt{x^2+1}}}{4(x^2+1)^{3/2}\bigl(\sqrt{x^2+1}+2x\bigr)},\]
可见 `f(x)` 在 `\bigl[0,\sqrt{1/3}\bigr]` 内下凸,在 `\bigl[\sqrt{1/3},+\infty\bigr)` 内上凸,易知 `f(x)` 在 `x=1` 处的切线方程为
\[h(x)=\frac{\sqrt{1+\sqrt2}}{2\sqrt2}(x-1)+\sqrt{1+\sqrt2},\]
因为 `1\in\bigl[\sqrt{1/3},+\infty\bigr)`,且不难证明 `f(0)<h(0)`,那么,根据《撸题集》第 5 页定理 1.1.1 可知
\[f(x)\leqslant h(x), \quad x\in[0,+\infty),\]
所以,若 `a_i\geqslant0` 且 `a_1+a_2+\cdots+a_n=n`,则有
\[\sum_{i=1}^nf(a_i)\leqslant\sum_{i=1}^nh(a_i)=\frac{\sqrt{1+\sqrt2}}{2\sqrt2}\left( \sum_{i=1}^na_i-n \right)+n\sqrt{1+\sqrt2}=n\sqrt{1+\sqrt2},\]
令 `n=2` 即得原题。 |
|