|
|
Last edited by hbghlyj 2023-8-28 20:50GR1268第11题
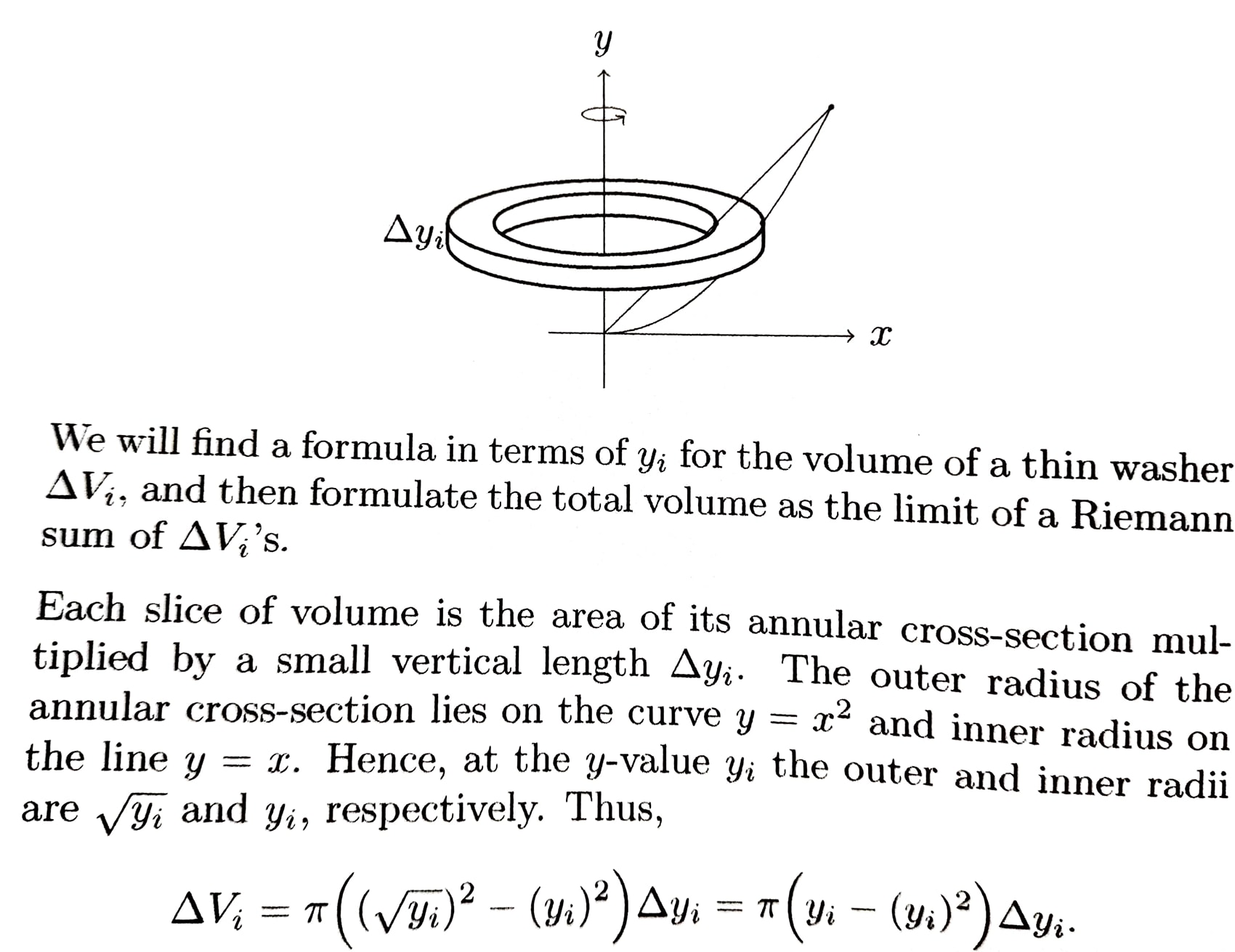
The region bounded by the curves $y=x$ and $y=x^2$ in the first quadrant of the $x y$-plane is rotated about the $y$-axis. The volume of the resulting solid of revolution is ___
答案:
We will find a formula in terms of $y_i$ for the volume of a thin washer $\Delta V_i$, and then formulate the total volume as the limit of a Riemann sum of $\Delta V_i$ 's.
Each slice of volume is the area of its annular cross-section multiplied by a small vertical length $\Delta y_i$. The outer radius of the annular cross-section lies on the curve $y=x^2$ and inner radius on the line $y=x$. Hence, at the $y$-value $y_i$ the outer and inner radii are $\sqrt{y_i}$ and $y_i$, respectively. Thus,
$$
\Delta V_i=\pi\left(\left(\sqrt{y_i}\right)^2-\left(y_i\right)^2\right) \Delta y_i=\pi\left(y_i-\left(y_i\right)^2\right) \Delta y_i
$$ |
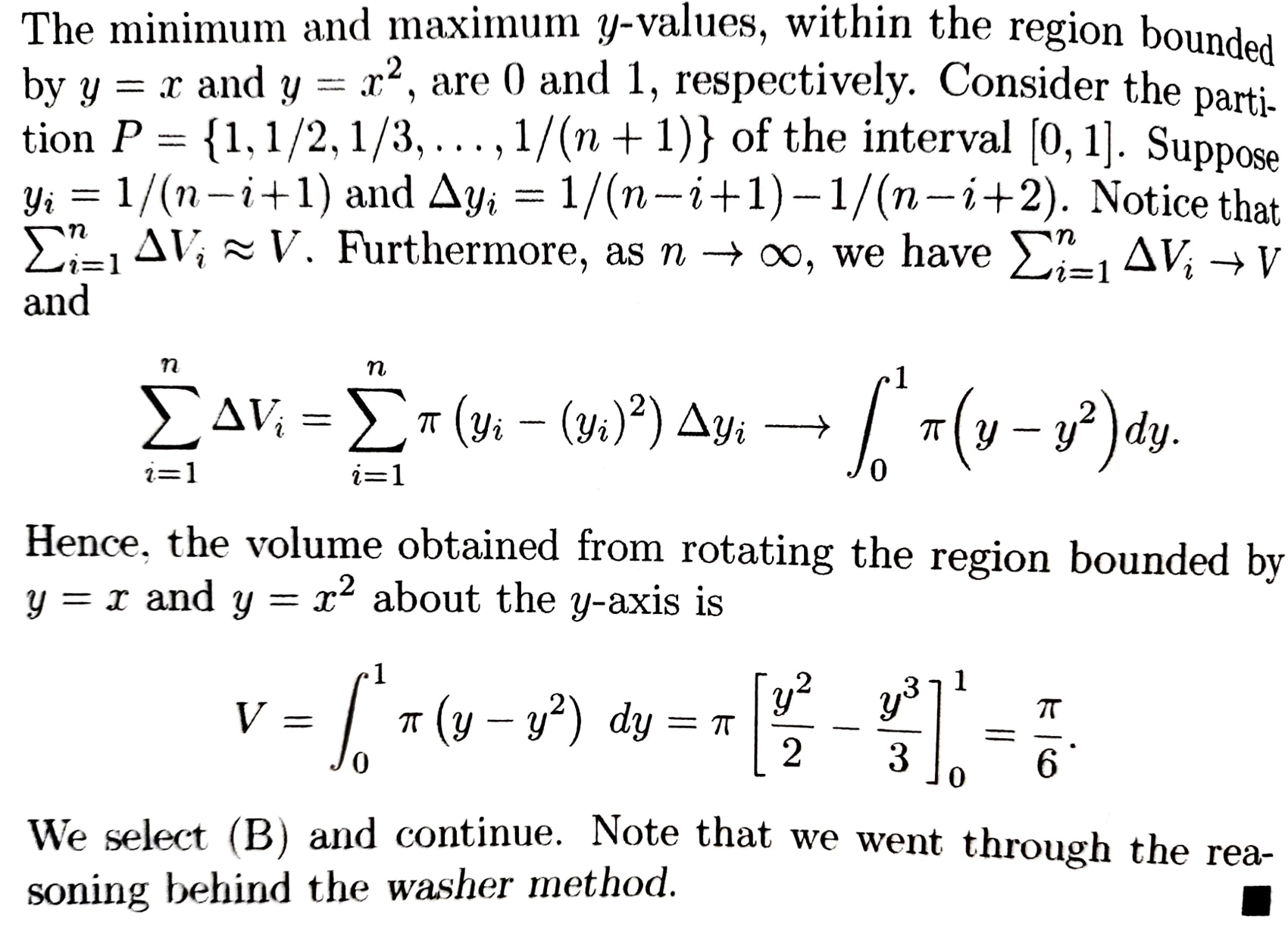
| The minimum and maximum $y$-values, within the region bounded by $y=x$ and $y=x^2$, are 0 and 1 , respectively. Consider the partition $P=\{1,1 / 2,1 / 3, \ldots, 1 /(n+1)\}$ of the interval $[0,1]$. Suppose $y_i=1 /(n-i+1)$ and $\Delta y_i=1 /(n-i+1)-1 /(n-i+2)$. Notice that $\sum_{i=1}^n \Delta V_i \approx V$. Furthermore, as $n \rightarrow \infty$, we have $\sum_{i=1}^n \Delta V_i \rightarrow V$ and
$$
\sum_{i=1}^n \Delta V_i=\sum_{i=1}^n \pi\left(y_i-\left(y_i\right)^2\right) \Delta y_i \longrightarrow \int_0^1 \pi\left(y-y^2\right) d y .
$$
Hence, the volume obtained from rotating the region bounded by $y=x$ and $y=x^2$ about the $y$-axis is
$$
V=\int_0^1 \pi\left(y-y^2\right) d y=\pi\left[\frac{y^2}{2}-\frac{y^3}{3}\right]_0^1=\frac{\pi}{6} .
$$We select (B) and continue. Note that we went through the reasoning behind the washer method. |
|
我的问题:$P=\{1,1 / 2,1 / 3, \ldots, 1 /(n+1)\}$是$[0,1]$的划分,但划分子区间长度最大值是$\frac12$不趋于0,所以它的黎曼和不趋于积分吧? |
|