求助：异面直线距离一公式

数学小黄

kuing

真难推，弄了我一个多小时。



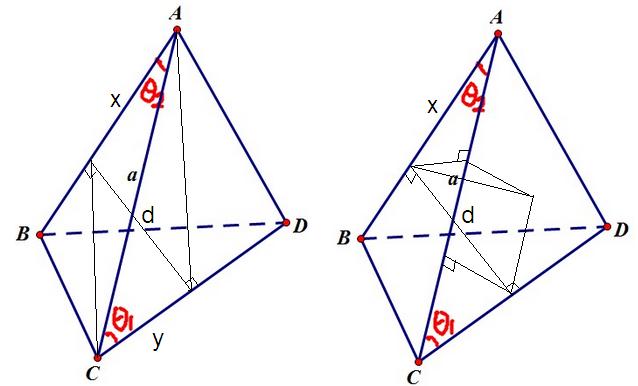
首先我们作出公垂线，然后连成如左图那样，由勾股定理及余弦定理可得
\begin{align*}
x^2+d^2&=a^2+y^2-2ay\cos\theta_1,\quad(1) \\
y^2+d^2&=a^2+x^2-2ax\cos\theta_2,\quad(2)
\end{align*}
再连成右图那样，又由勾股定理及余弦定理可得
\begin{align*}
d^2&=(a-y\cos\theta_1-x\cos\theta_2)^2+(y\sin\theta_1)^2+(x\sin\theta_2)^2-2xy\sin\theta_1\sin\theta_2\cos\alpha\\
&=a^2+x^2+y^2-2a(y\cos\theta_1+x\cos\theta_2)+2xy\cos\theta_1\cos\theta_2-2xy\sin\theta_1\sin\theta_2\cos\alpha,\quad(3)
\end{align*}
将式 (3) 分别代入式 (1), (2) 化简整理可得
\begin{align*}
x+(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)y&=a\cos\theta_2, \\
y+(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)x&=a\cos\theta_1,
\end{align*}
于是解得
\begin{align*}
x&=\frac{a\cos\theta_2-a\cos\theta_1(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)}{1-(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)^2},\\
y&=\frac{a\cos\theta_1-a\cos\theta_2(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)}{1-(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)^2},
\end{align*}
将式 (1), (2) 相加并代入上述解可得
\begin{align*}
d^2={}&a^2-ay\cos\theta_1-ax\cos\theta_2 \\
={}&a^2-\frac{a^2\cos^2\theta_1-a^2\cos\theta_1\cos\theta_2(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)}{1-(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)^2} \\
&{}-\frac{a^2\cos^2\theta_2-a^2\cos\theta_1\cos\theta_2(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)}{1-(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)^2} \\
={}&a^2\left( 1-\frac{\cos^2\theta_1+\cos^2\theta_2-2\cos\theta_1\cos\theta_2(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)}{1-(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)^2} \right) \\
={}&a^2\cdot \frac{1-\sin^2\theta_1\sin^2\theta_2\cos^2\alpha -\cos^2\theta_1-\cos^2\theta_2+\cos^2\theta_1\cos^2\theta_2}{1-(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)^2} \\
={}&a^2\cdot \frac{\sin^2\theta_1\sin^2\theta_2-\sin^2\theta_1\sin^2\theta_2\cos^2\alpha }{(\sin^2\theta_1+\cos^2\theta_1)(\sin^2\theta_2+\cos^2\theta_2)-(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)^2} \\
={}&\frac{a^2\sin^2\theta_1\sin^2\theta_2\sin^2\alpha }{\sin^2\theta_1\sin^2\theta_2\sin^2\alpha +\sin^2\theta_1\cos^2\theta_2+\sin^2\theta_2\cos^2\theta_1+2\cos\theta_1\cos\theta_2\sin\theta_1\sin\theta_2\cos\alpha} \\
={}&\frac{a^2\sin^2\alpha }{\sin^2\alpha +\cot^2\theta_2+\cot^2\theta_1+2\cot\theta_1\cot\theta_2\cos\alpha},
\end{align*}
即得证。

数学小黄

回复 2# kuing
神啊~膜拜管理员！！太感激您了~

kuing

回复 3# 数学小黄

不客气，我可能搞复杂了也说不准，有空再想想有没有更简单的证法

福州小江

KK神级人物！！！！

睡神

不知道将它补成长方体有没有什么辅助效果不？

kuing

不知道将它补成长方体有没有什么辅助效果不？
睡神 发表于 2013-9-9 20:56

又没说对边相等，怎么确定能补成长方体？

睡神

额...好吧...糗大了...

福州小江

回复 4# kuing


    记得楼主在另一个群里说这题可以无字证明！不知道是不是真的？

kuing

回复 9# 福州小江

噢？那就真值得期待

其妙

回复  kuing


    记得楼主在另一个群里说这题可以无字证明！不知道是不是真的？ ...
福州小江 发表于 2013-9-9 21:34

认识楼主啊？

福州小江

回复 11# 其妙


    楼主在齐老师的群里问了相同的问题！只是加了无字证明的要求！！！

kuing

原来只是个要求？

第一章

数学小黄 发表于 2013-9-9 10:43

以前做过类似的，貌似转化为点到面的距离。如，过$C$作$AB$的平行线，并截取相等。

kuing

有简单点的证法了。

首先证明一个公式，如左图，$\angle AOB=\theta_1$, $\angle AOC=\theta_2$, $\text{二面角}~B\text{-}OA\text{-}C=\beta$，我们来求 $\cos\angle BOC$。

在射线 $OA$ 上取一个单位长度，然后作垂直，连成左图中那样，由余弦定理即得
\begin{align*}
\cos\angle BOC &=\frac{\sec^2\theta_1+\sec^2\theta_2-(\tan^2\theta_1+\tan^2\theta_2-2\tan \theta_1\tan\theta_2\cos\beta)}{2\sec\theta_1\sec\theta_2} \\
&=\cos\theta_1\cos\theta_2(1+\tan\theta_1\tan\theta_2\cos\beta) \\
&=\cos\theta_1\cos\theta_2+\sin\theta_1\sin\theta_2\cos\beta.
\end{align*}



回到原题，设四面体 $ABCD$ 的体积为 $V$，$AB=b$, $CD=c$ 且 $AB$ 与 $CD$ 所成夹角为 $\theta$，则易证
\[V=\frac{bcd\sin\theta}6,\]
另一方面，$S_{\triangle ABC}=\frac12ab\sin\theta_2$，易知 $D$ 到 $\triangle ABC$ 所在平面的距离为 $c\sin\theta_1\sin\alpha$，所以又有
\[V=\frac13\cdot\frac12ab\sin\theta_2\cdot c\sin\theta_1\sin\alpha=\frac{abc\sin\theta_1\sin\theta_2\sin\alpha}6,\]
由此得到
\[d\sin\theta=a\sin\theta_1\sin\theta_2\sin\alpha,\]
故剩下只要求 $\sin\theta$，如右图，将 $AB$ 平移到 $CE$，那么 $\angle ACE=\theta_2$, $\angle ECD=\theta$, $\text{二面角}~E\text{-}AC\text{-}D=\pi-\alpha$，根据前面所证得的公式，即得
\[\sin\theta=\sqrt{1-\cos^2\theta} =\sqrt{1-(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)^2},\]
所以
\[d=\frac{a\sin\theta_1\sin\theta_2\sin\alpha}{\sqrt{1-(\cos\theta_1\cos\theta_2-\sin\theta_1\sin\theta_2\cos\alpha)^2}},\]
然后跟我2楼后面那个化简方法一样，得到最终的公式。

kuing

唔，其实严格来说，还应该要说明一下那公式对 $\theta_1$ 或 $\theta_2$ 为钝角时仍然成立，不过这个应该不难说明，时间关系还是先略去了……

realnumber

用向量不知道行不行，给的条件似乎都可以用向量直接描述出来，不过叉积运算性质不清楚.

爪机专用

用向量不知道行不行，给的条件似乎都可以用向量直接描述出来，不过叉积运算性质不清楚. ...
realnumber 发表于 2013-9-11 10:17

我也考虑过, 不过向量我也不擅长, 不知怎么消。
电脑又上不了论坛, 爪机却可以, 汗。

其妙

异面直线的夹角就可以向量。距离似乎可以，但是那个条件不好用，

数学小黄

回复 9# 福州小江

啊？应该不是我说的吧，我是在一本书上看到这个结论，书的作者是当结论直接给出了，我思来想去都不知道为什么，所以才拿到这里请教大家。