有理点 单位圆内接五角星面积为1

hbghlyj

单位圆内接五角星$ABCDE$的面积为1,$A(1,0),B,D$在上半平面且$\angle BOD=90^\circ$,作$B,D$关于$x$轴的对称点$E,C$,求所有的有理点$B$.

敬畏数学

回复 3# hbghlyj
你是学生，做这么难的题？

hbghlyj

$B(-\frac35,\frac45),C(\frac45,-\frac35),D(\frac45,\frac35),E(-\frac35,-\frac45)$
GeoGebra按代数规则计算自交多边形的面积.中间的五边形,缠绕数为2,所以面积算2次;周围的5个小三角形,缠绕数为1,所以面积算1次.(但是,它显示的中间的凸五边形是白色的,与它的面积计算规则相悖)
$\frac12\left(1\cdot\frac45-0\cdot\left(-\frac35\right)+\left(-\frac35\right)^2-(\frac45)^2+\frac45\cdot\frac35-\left(-\frac35\right)\cdot\frac45+\frac45\cdot\left(-\frac45\right)-\frac35\left(-\frac35\right)+\left(-\frac35\right)\cdot0-\left(-\frac45\right)\cdot1\right)=1$
在mathematica12中,polygon的内部是按奇偶规则计算的.中间的五边形,缠绕数为2,所以面积算0次;周围的5个小三角形,缠绕数为1,所以面积算1次.
poly=Polygon[{{1, 0}, {-3/5, 4/5}, {4/5, -3/5}, {4/5, 3/5}, {-3/5, -4/5}}];
Show[Graphics[{Circle[],poly}]];

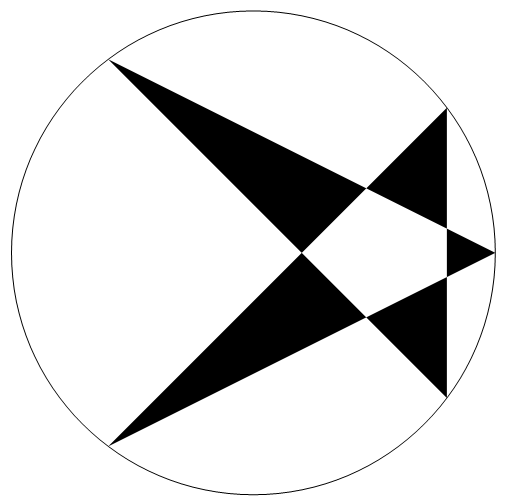
Area[poly]
输出$\frac{46}{75}$
Area[OuterPolygon[poly]]+Area[InnerPolygon[poly]]
输出1.这样就与GeoGebra计算得一样了.都是中间和凸五边形算2次.

hbghlyj

设单位圆上的有理点B为$\left(\frac{b^2-a^2}{a^2+b^2},\frac{2 a b}{a^2+b^2}\right),$其中a,b是互质的正整数.
按照代数规则计算出的五边形面积为$A=\frac{-6 a^2 b^2+4 a^3 b+a^4+b^4}{\left(a^2+b^2\right)^2}$.
令A=1得$4 a^2 b (a-2 b)=0$,所以a=2,b=1.
所以仅有此一组解.